How do you find the roots of a cubic equation?

How do you find the roots of a cubic equation?

How do you find the cubic equation on a calculator?

The procedure to use the cubic equation solver calculator is as follows:

1. Step 1: Enter the equation in the respective input field.
2. Step 2: Now click the button “Solve” to get the variable value.
3. Step 3: Finally, the result of cubic equation will be displayed in the new window.

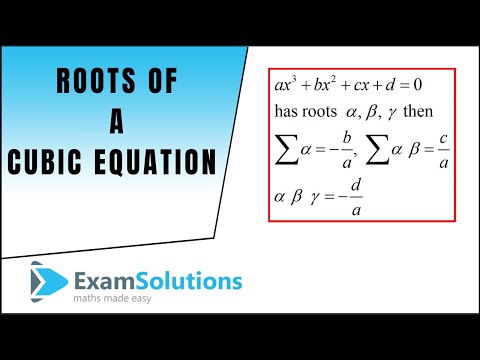
What are the 3 roots of cubic equation?

The three roots of x3 + ax + b are the real numbers 2R, -R + /3I, and -R – /3I. These four steps together are the cubic formula. It uses complex numbers (D and z) to create real numbers (2R, -R + /3I, and -R – /3I) that are roots of the cubic polynomial x3 + ax + b.

How do you find roots?

For a quadratic equation ax2 + bx + c = 0, The roots are calculated using the formula, x = (-b ± √ (b² – 4ac) )/2a. Discriminant is, D = b2 – 4ac. If D > 0, then the equation has two real and distinct roots.

What is the sum of roots of a cubic equation?

Sum of the roots = −b/a = -b. Product of the roots = c/a = c.

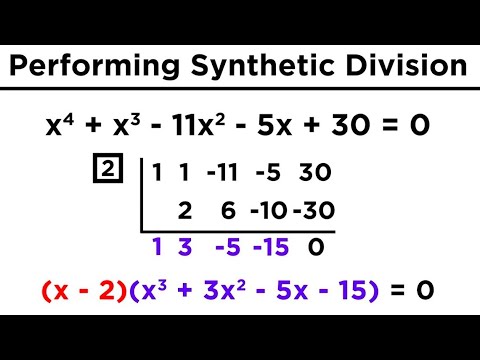
How do you find the roots of a cubic equation using synthetic division?

How do you simplify a cubic equation?

What is the easiest way to find the roots of a quadratic equation?

Why do we find roots of equations?

This is because: it was discovered that equations we are interested in solving can be transformed into equivalent equations where one side is zero. So if we can solve that case, then we can solve other cases, too!

How do you solve cubic equations without a calculator?

All you need to do is use the factoring approach, only first you must add 2 to both sides. After, you can factor it to (x) (x^2 + 4) = 2. Divide both sides by x to get x^2 + 4 = 2/x. Subtract 4 from both sides to get x^2 = 2/x – 4.

How do you solve a cubic equation with complex roots?

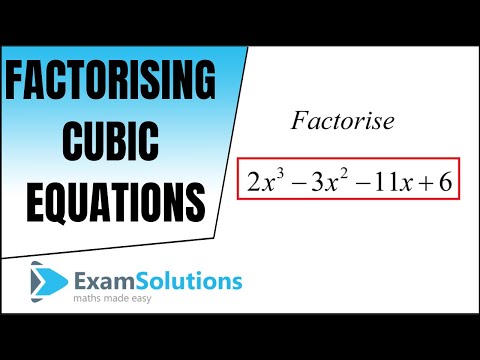
How do you Factorise cubics quickly?

Factorising Cubic Polynomial

1. Find x = a where p(a) = 0.
2. Then (x – a) is the factor of p(x)
3. Now divide p(x) by (x – a) i.e. (p(x))/((x – a))
4. And then we factorise the quotient by splitting the middle term.